Variable and Random Variable – continuous case

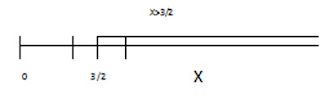
Let us consider the inequality 2X > 3 …….(1). This inequality is satisfied by X > 3/2, so here {X|X>3/2}. So the solution set of (1) is an infinite set and lies in a continuum. It can be represented as 

Whereas for,

 One Dimensional Random Variables

We illustrate with the following example.

Let X be a random variable denoting time taken by an individual in a telephone call. The PDF can be explained by Exponential Distribution with parameter λ..

Now let’s consider the following inequalities.

2X+3Y< 5 and X-3Y<2, the solution to these inequalities can be represented by the shaded region in the following diagram

 A Two Dimensional Random Variable

Let X be a random variable denoting the time of failure of an electronic device and Let Y be a random variable denoting the time to repair this device.

We assume X and Y to be independent.

Then {(X. Y) |X>0, Y>0}. The PDF of X can be represented by Exponential Distribution and Y Can be represented by Normal Distribution.